LeetCode 563 - Binary Tree Tilt (Easy)

Given the root of a binary tree, return the sum of every tree node's tilt.

The tilt of a tree node is the absolute difference between the sum of all left subtree node values and all right subtree node values. If a node does not have a left child, then the sum of the left subtree node values is treated as 0. The rule is similar if there the node does not have a right child.

 

Example 1:

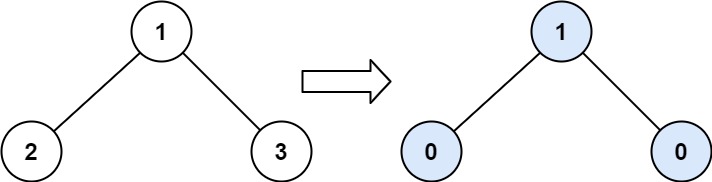
Input: root = [1,2,3]
Output: 1
Explanation: 
Tilt of node 2 : |0-0| = 0 (no children)
Tilt of node 3 : |0-0| = 0 (no children)
Tile of node 1 : |2-3| = 1 (left subtree is just left child, so sum is 2; right subtree is just right child, so sum is 3)
Sum of every tilt : 0 + 0 + 1 = 1

Example 2:

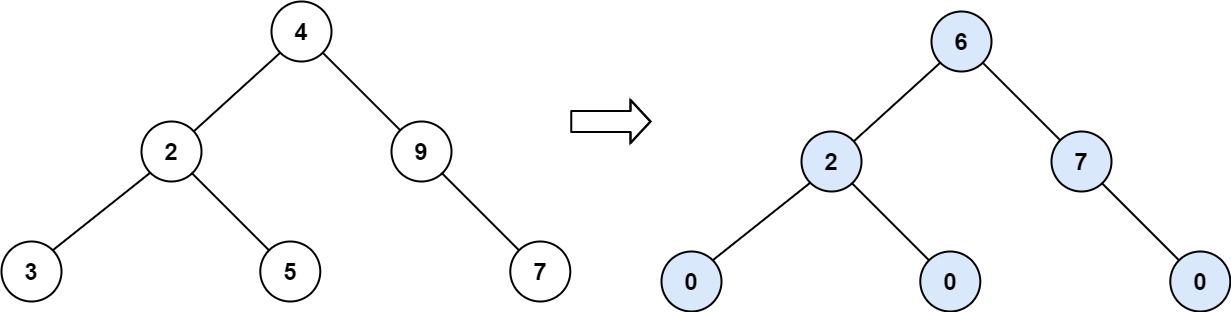
Input: root = [4,2,9,3,5,null,7]
Output: 15
Explanation: 
Tilt of node 3 : |0-0| = 0 (no children)
Tilt of node 5 : |0-0| = 0 (no children)
Tilt of node 7 : |0-0| = 0 (no children)
Tilt of node 2 : |3-5| = 2 (left subtree is just left child, so sum is 3; right subtree is just right child, so sum is 5)
Tilt of node 9 : |0-7| = 7 (no left child, so sum is 0; right subtree is just right child, so sum is 7)
Tilt of node 4 : |(3+5+2)-(9+7)| = |10-16| = 6 (left subtree values are 3, 5, and 2, which sums to 10; right subtree values are 9 and 7, which sums to 16)
Sum of every tilt : 0 + 0 + 0 + 2 + 7 + 6 = 15

Example 3:

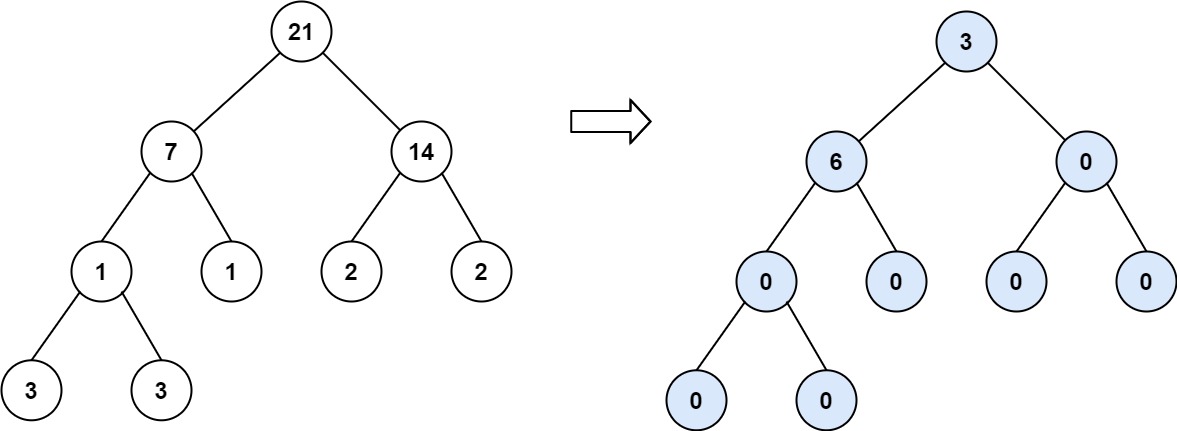
Input: root = [21,7,14,1,1,2,2,3,3]
Output: 9

 

Constraints:

• The number of nodes in the tree is in the range [0, 104].
• -1000 <= Node.val <= 1000

 方法：dfs 思路：当选择dfs的时候，有三种遍历方式，pre-order，in-order，post-order。在这一题里，我们需要分别先得到左右节点的信息，然后是根节点的信息。所以post-order会是 更加合适的一种遍历方式。对每一个节点而言，tilt = abs(sum of node.left - sum of node.right), total_tilt += tilt。那么在函数中返回上一层节点的信息会是什么呢？是下层节点的 sum，所以我们返回sum of node.left + sum of node.right + node.val  time complexity: O(n)  sp

# Definition for a binary tree node.
# class TreeNode:
#     def __init__(self, val=0, left=None, right=None):
#         self.val = val
#         self.left = left
#         self.right = right
class Solution:
    def findTilt(self, root: TreeNode) -> int:
        if not root: return 0 
        self.res = 0 
        self.postOrder(root)
        return self.res 
    
    def postOrder(self, root):
        if not root: return 0 
        left = self.postOrder(root.left)
        right = self.postOrder(root.right)
        self.res += abs(left - right)
        return left + right + root.val

 

ace complexity: O(n)   

标签：node,Binary,right,Tilt,sum,563,root,self,left
来源： https://www.cnblogs.com/sky37/p/13946703.html